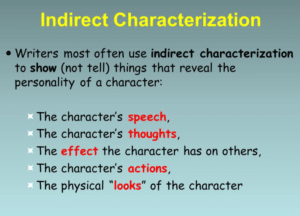
Set notation defines the elements and also properties of sets utilizing symbols. Symbols save you room when creating and also describing sets.
Set notation likewise helps us to explain various partnerships between 2 or even more sets utilizing icons. In this manner, we can easily carry out operations on sets, such as intersections and unions.
You can never inform when set notation will undoubtedly show up, and it can be in your algebra course! Therefore, understanding the icons utilized in the set concept is an asset.
In this short article, you will indeed find out the following:
Exactly how to specify a set notation
Just how to read and write set notation
You will find a brief quiz and a response trick at the end of this article. Remember to evaluate just how much you’ve understood.
Let’s begin with the definition of set notation.
Understanding more about set notation
Set notation is a system of icons made use of:
define aspects of a set
show connections amongst sets
show operations amongst sets
In the previous post, we used some symbols when explaining sets. Do you understand the characters displayed in the table below?
∉ ‘is not an element of or ‘is not a member of
∈ ‘is an element of or ‘is a member of
|
‘such that’ or ‘for which
{ } denotes a set
: ‘such that’ or ‘for which
How do we check out and also create a set notation?
To review as well as write set notation, we need to comprehend how to make use of icons in adhering to cases:
1. Denoting a Set
Conventionally, we signify a set by a capital letter and represent the components of the set by lower-case letters.
We usually separate the elements utilizing commas.
We read this as ‘the set A having the vowels of the English alphabet’.
2. Set Membership
We use the symbol ∈ is utilized to denote Membership in a set.
Given that 1 is an aspect of set B, we compose one ∈ B and review it as ‘1 is a component of set B’ or ‘1 is a part of set A’.
Because 6 is not an aspect of set B, we write six ∉ B and review it as ‘6 is not a component of set B’ or ‘6 is not a part of set B’.
3. Defining the Members of a Set
In the previous explanation describing sets, we applied set notation to explain sets. Please keep in mind the set-builder notation!
4. Subsets of a set
We assume that A-set A is a subset of B-set B when all component of A is additionally an element of B. A is contained in B.
5. Correct Subsets of a Set
We state that set A is an appropriate subset of set B when every aspect of A is additionally an element of B. However, at the very least, a component of B is not in A.
6. Equal Sets
If all the elements of set A become a component of set B, and every component of B is likewise an aspect of A, then we state that set A is equal to set B.
We utilize the notation below to show that the two sets are equivalent.
7. The Empty Set
It is a set that has no aspects. We can likewise call it a null set. We recognize the empty set by the sign ∅ or by empty curly braces,
Remembering that the empty set is a subset of every set.
8. Singleton
It includes precisely one component. Because of this factor, we also call it a unit set. As an example, the set comprises just one component,1.
We confine the single component in curly braces to signify a singleton.
9. The Universal Set
The universal set is a set which contains all the components present. Traditionally, we use the icon U to represent the universal set.
10. The Power Set
The power set includes all the subsets of A. We represent a power set by P(A) and consider it as ‘the power set of A.’
11. The Union of Sets
The union of set An and set B is the set which contains all aspects in set B or set A or in both set An and also set B.
We denote An and B’s union by A ⋃ B and review it as ‘A union B.’ We can likewise use the set-builder notation to define An and B’s union.
The union of three or even more sets includes all the components in each of the sets.
An aspect comes from the union if it belongs to a minimum of one of the sets.
12. Set Difference
The set difference between set An and set B is the set of combined elements found in A yet not in B.
We denote An and B’s set difference by A-B or A \ B and read it as ‘A difference B.’
The set difference between An and B is called the family member enhancement of B concerning A.
Read Also:45°-45°-90° Triangle Explained with Examples