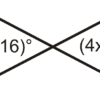
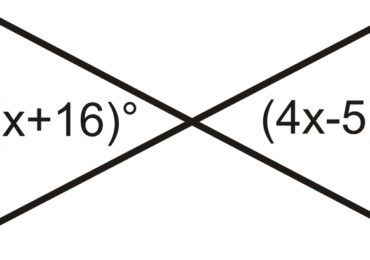Since you understand a triangle is a two-dimensional polygon with three sides, three angles, and three vertices. This article is most likely to find out other types of triangles known as special right triangles. Before we can start, let’s recall the best triangle.
About Special Right triangles
The term “best” refers to the Latin word “rectus,” suggesting upright. As a result, the best triangle is a triangle whose one angle is 90 levels (right angle). The right triangle is indicated with a box at the place of the right angle.
The lengthiest side of the right triangle on the opposite side of the best angle is called the hypotenuse. The two other sides of the triangle are called legs. The straight leg is the base, and also the upright leg is the height of the aright triangle.
Details on Special Right Triangles
Special right triangles are triangles whose sides remain in a particular proportion, referred to as Pythagorean Triples. In geometry, the Pythagorean Theory is a declaration that shows the partnership of the sides of the best triangle.
A right triangle formula is provided by a2 + b2 = c2, where either b or a is the triangle’s base and height. And c is the hypotenuse. By utilizing the Pythagorean Thesis, discovering the absent side of a triangle is relatively easy and straightforward.
Both Special right triangles include:
45 °; 45 °; 90 ° Triangle
30 °; 60 °; 90 ° Triangle
Let’s have a short introduction of these special right triangles as we will see them thoroughly in the next articles.
The 45 °; 45 °; 90 ° Triangle. In particular, the right triangle angles are 45 °, 45 °, and also 90 °. The proportion of the base to height to this triangle’s hypotenuse is 1: 1: √ 2.
Now, you can see – Base: Height: Hypotenuse = x: x: x √ 2 = 1: 1: √ 2.
Alternatively, a 45 °; 45 °; 90 ° triangle can additionally be an isosceles triangle. An isosceles triangle is a triangle in which two the lengths of its two sides are equal, and likewise, both of its angles are equal.
By utilizing the formula of a special right triangle a2 + b2 = c2, we can determine the hypotenuse of a 45 °; 45 °; 90 ° triangle as complies with: Because a 45 °; 45 °; 90 ° triangle is additionally an isosceles triangle; let a
= b =x; x2
+ x2= 2×2 Locate the square root of each term in the formula √ x2 + √ x2 = √( 2×2).
x + x = x √ 2.
For that reason, the hypotenuse of a 45 °; 45 °; 90 ° triangle is x √
- The 30 °; 60 °; 90 ° Triangle.
This is a special angle triangle whose angles are 30 °; 60 °; 90 °. The proportion of the lengths of the sides are x: x √ 3: 2x.
Read Also: What Is Indirect Characterization? Examples