Calculus Calculator: The fundamental theorem of calculus says that if f(x) is constant between an and also b, the indispensable from x=a to x=b off(x)dx is equal to F(b) – F(a), where the derivative of F concerning x amounts to f(x). The big F is what’s called an anti-derivative of little f. This is one of the most critical points in all of mathematics, and it’s called the fundamental thesis of calculus. Yet what the heck does ‘essential’ indicate?
Consider a chart of velocity as a function of time – so this is f(t) – between some point in time a, and also some point in time b. Let’s state that f(t) is constant in between an and b, to make things essential. I recognize that, according to the basic theory of calculus, the integral from a to b of’ f(t)dt – so that’s the area under this contour – is equal to F(b) – F(a), where F'( t) = f (t). That’s the essential theorem of calculus. Yet let’s consider it.
Calculus Calculator – Working Methodology
The calculator on this page calculates your dual essential symbolically by using a computer system algebra system. In symbolic integration, the computer makes use of algebra and also policies critical to take the antiderivative of the feature before applying the fundamental thesis of calculus. In essence, symbolic combination complies with the same steps as a human with a paper and pencil would. It can acquire near-ideal service accuracy. The calculator on this web page is precise to a minimum of the 5th decimal place!
Read Also: What Are The Agents Of Socialization? Example
The choice of utilizing symbolic integration to fix integrals is called numerical methods/integration. A mathematical regular executes a relatively small, approximated variation of the trouble as sometimes as necessary to merge to a perfect remedy. Usually, mathematical routines can fix a more excellent range of issues yet can take longer and also possibly be less accurate.
Essential Theory in Practice
F(t) is equal to f as well as the by-product of 30t is similar to 30
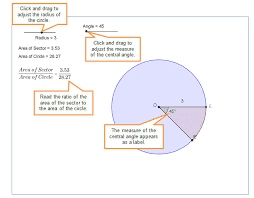
Fundamental Theorem Example Graph
Let’s claim f(t)=30 miles per hour (miles per hour). Let’s first locate an F that you can take the by-product of to get 30. So here I intend to find what’s called an anti-derivative off. Let’s state that F=30t. If I take the derivative of 30t, I get d/dt(30t), which amounts to 30d/dt(t), which is just 30. So today, I understand that F'(t)=f, as well as the by-product of 30t, is equal to 30, so the derivative of this is similar to this.
Okay, so currently I’ve got f(t), which is 30, and also my anti-derivative here is 30t, so allow plug those in. Let’s say I’ve obtained my speed right here, 30, and I’m integrating it between an and b. According to the fundamental theorem, this amounts to F(b), so F, which is 30t, assessed at t=b, so that amounts to 30b – F(a), which is 30a. So, according to the fundamental theory of calculus, the essential from a to b of 30dt=30b – 30a. I can simplify this right-hand side to equivalent 30(b – a).
Read Also: What Is Bass Clef Notes?
Well, let’s take a look at our graph. Our graph is just a straight line, so the essential in this situation is simply this rectangle right here. Well, the area of a rectangular shape is the height times the size. My elevation is 30, because f(t)=30. My width is b – a. So certainly, that’s what this right-hand side amounts to. It equals my height times my width, which is specifically the location.
About Calculus eBooks
For the majority of us, learning calculus isn’t very easy. How many times have you looked up the response to a problem in the back of the book? And smacked yourself on the head claiming, “how did they get that”?
Well, those days more than. A brand-new calculus e-book called “Calculus without Limits” approaches the subject in various way-showing you in a detailed step-by-step style of how to do calculus problems. Conversations of theses, as well as proofs, are avoided for a plain-English strategy based on trouble solving.
Read Also: What Is Vertex Form? Example
The book covers most initial year university calculus programs. Introductory chapters include limits, derivatives, and also the applications of the derivative. However, the book is called “Calculus without Limits,” that’s simply a pun, as calculating limits are covered carefully. Solved instances consist of standard restriction computation, one-sided limitations, unlimited restrictions, and limits at infinity. Limitations of trig functions, exponentials, and logarithms additionally included.
Two phases of distinction are extensive. The first one sets out the basics of computing derivatives like the power rule and derivative of a consistent; after that, it is on to the first regulations every person struggles with, the item rule, the ratio guideline, and the chain regulation. The following phase, applications of the derivative, covers standard subjects like max-min troubles, Rolle’s theory, as well as implicit differentiation, together with computing derivatives of the inverted trig and also hyperbolic functions.
Double Integrals In-Detail
As we can see in the picture over, z = f(x, y) is a feature of x and y, and for that reason, the function produces a surface area with z values that vary with x as well as the y. The area is a rectangular shape with side lengths identified by the size of the integrating area for x and also y. There is an excellent variety of infinitesimal columns that expand from the surface area down to the x-y aircraft. These extremely tiny columns notated as dxdy or dydx depending upon the order of integration we select when setting the issue up. The necessary double calculator on this page uses the order dxdy because it streamlines your inputs.
When calculating a double important by hand, we can pick either dxdy or dydx because either will undoubtedly obtain the appropriate option. We must ensure that the order of the critical limits matches the request of dxdy or dydx. This is since we do a dual integral sequentially, from inside to outside. As a result, x limits needed throughout the dx section of the essential, and the y limits ought to be made use of during the dy portion of the integral.