Centripetal Force Equation
Centripetal Force Equation: Do you remember riding on the merry-go-round as a kid? Did you ever stand at the very edge of the merry-go-round and hold on tight to the railing as your friends pushed the wheel faster and faster? Maybe you remember that the faster the wheel turned, the harder it became to hold on. You might not have known it at the time, but you were creating a balance between two forces – one real and one apparent – in order to stay on that circular path.
Merry-go-rounds are a perfect example of how a force is used to keep an object moving in a circular path. Your body wanted to fly off the merry-go-round in a straight line, but your hands exerted an opposing force to keep you on. The tendency for your body to fly off the merry-go-round is called centrifugal force. It isn’t a real force, but an apparent one. The force you used with your hands to stay on the ride is real, and it is called the centripetal force. Let’s learn more about it.
 Centripetal Force And Centripetal Acceleration
Centripetal Force And Centripetal Acceleration
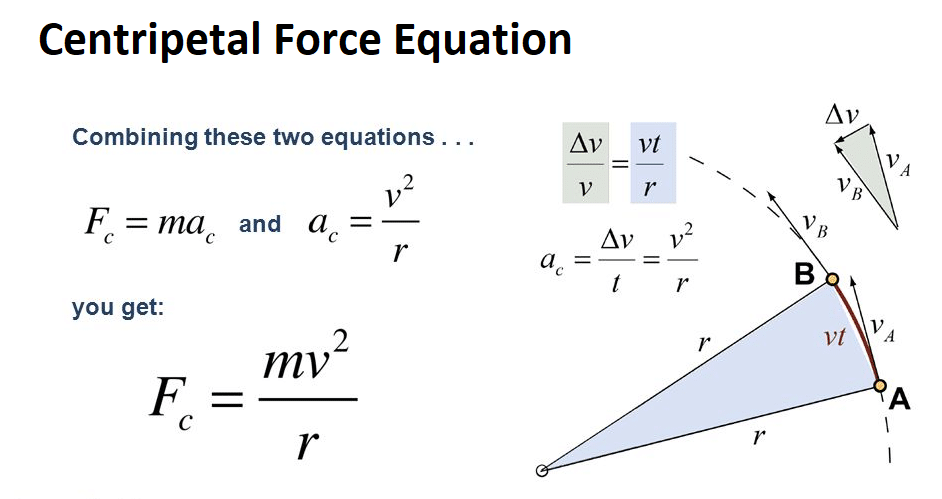
Centripetal acceleration is the name for the acceleration directly toward the center of the circle in a circular motion. This is defined by:
a = v2 / r
Where v is the speed of the object in the line tangential to the circle, and r is the radius of the circle it is moving in. Think about what would happen if you were swinging a ball connected to a string in a circle, but the string broke. The ball would fly off in a straight line from its position on the circle at the time the string broke, and this gives you an idea of what v means in the above equation.
Because Newton’s second law states that force = mass × acceleration, and we have an equation for acceleration above, the centripetal force must be:
F = mv2 / r
In this equation, m refers to a mass.
So, to find the centripetal force, you need to know the mass of the object, the radius of the circle it’s traveling in, and its tangential speed. Use the equation above to find the force based on these factors. Square the speed, multiply it by the mass and then divide the result by the radius of the circle.
Read Also: Antiderivative Calculator – A Brief Introduction
Centripetal Force With Incomplete Information
If you don’t have all the information you need for the equation above, it might seem like finding the centripetal force is impossible. However, if you think about the situation, you can often work out what the force might be.
For example, if you’re trying to find the centripetal force acting on a planet orbiting a star or a moon orbiting a planet, you know that the centripetal force comes from gravity. This means you can find the centripetal force without the tangential velocity by using the ordinary equation for gravitational force:
F = Gm1m2 / r2
Where m1 and m2 are the masses, G is the gravitational constant, and r is the separation between the two masses.
To calculate centripetal force without a radius, you need either more information (the circumference of the circle related to radius by C = 2π_r, for example) or the value for the centripetal acceleration. If you know the centripetal acceleration, you can calculate the centripetal force directly using Newton’s second law, F = ma.
Read Also: What Is A Concentration Gradient? Defination